Why Compartmentalising Is A Bad Idea When Teaching Mathematics
Most skills are best taught compartmentalised - i.e., in progressive parts.
An effective way to teach someone to throw a ball (for example, an outfield throw in baseball or cricket) is to have the person:
A similar principle applies when teaching students to write stories. There is no point asking a child to write a ten-page story if they haven’t progressed from writing words to sentences to paragraphs, and then to stories 2-3 pages long. And, of course, along the way, learning the grammar. What about school mathematics?
The field of school mathematics can be divided into hundreds of components that build upon each other. Therefore, it is logical to assume that we need to compartmentalise mathematics when teaching it. Clearly, compartmentalisation has been integral to mathematics education since we first started teaching the subject en masse.
|
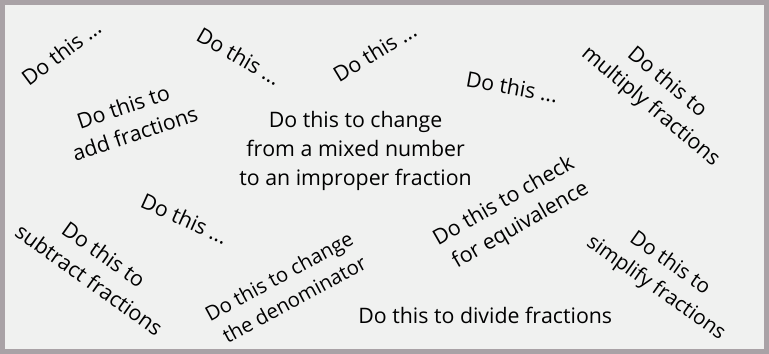
Compartmentalised maths teaching in action ...
We all understand what compartmentalised mathematics teaching looks like. But to help drive the point home, consider a fractions, decimals and percentages example ...
- First, make sure you teach all three as distinct topics despite the fact they are all based on the same part-vs-whole concept.
- Then, within each topic (for example, fractions) compartmentalise by teaching the following skills in isolation:
- Simplifying fractions.
- Working with equivalent fractions.
- Converting between mixed numbers and improper fractions.
- Adding fractions with common denominators.
- Subtracting fractions with common denominators.
- Adding fractions with mixed denominators.
- Subtracting fractions with mixed denominators.
- Multiplying fractions.
- Dividing fractions.
- Calculating fractions of quantities.
- Using fractions in real-world situations
- And on and on it goes … (whew!)
The danger of over-compartmentalising
Compartmentalisating is ideal for the learning of physical skills. However, in some fields of learning, there is the danger of over-compartmentalising.
For example, when teaching writing, there’s a place for explicitly teaching grammar and for explicitly teaching spelling. But if we have lessons that are solely focused on grammar or solely focused on spelling, the learning becomes dull and we’ll likely turn the students off writing.
Surely, the best way to have students become good writers is to find something that they are passionate about and have them write about it. And then provide feedback on the better aspects of their writing. And along the way, support them in improving their spelling and grammar. This is far from a compartmentalised approach. The #1 aim, though, is to have students ENJOY writing.
For example, when teaching writing, there’s a place for explicitly teaching grammar and for explicitly teaching spelling. But if we have lessons that are solely focused on grammar or solely focused on spelling, the learning becomes dull and we’ll likely turn the students off writing.
Surely, the best way to have students become good writers is to find something that they are passionate about and have them write about it. And then provide feedback on the better aspects of their writing. And along the way, support them in improving their spelling and grammar. This is far from a compartmentalised approach. The #1 aim, though, is to have students ENJOY writing.
To compartmentalise … or to NOT compartmentalise mathematics. THAT is the question!
Again, it seems logical that compartmentalised approaches like those listed above would help the learning of mathematics. Surely, through compartmentalisation, we’ll allow students to gain success on each little task as they progress through the many tasks within a unit of work.
But there’s a problem …
However, there is a downside to the compartmentalised learning of mathematics. It turns out that learning trigonometry, straight-line graphing and fractions-decimals-percentages via a compartmentalised approach is MUCH MORE difficult for students than when learning in a more unified, less compartmentalised way. The same applies to the learning of most mathematics topics.
How can compartmentalising mathematics possibly HINDER the learning of mathematics?
I am not for a minute, suggesting we have students explore complicated, real-world problems when first presenting trigonometry.
Nor am I suggesting we have students try to determine the equations of difficult straight lines before they have worked with gradients and ordered pairs.
Nor am I suggesting there is NO place for compartmentalising when teaching mathematics.
What I am suggesting, and with conviction, is that the traditional, compartmentalised approach, by default, makes the learning of mathematics more difficult than it needs to be. I’m suggesting that, by default, compartmentalising the teaching of mathematics has more students spending more time in more lessons NOT understanding the work they have been tasked to complete - compared to a less-compartmentalised approach.
To appreciate why compartmentalising complicates the learning of mathematics - rather than making it more accessible - one needs to experience the alternative. The alternative is to present mathematics in a more unified way, a way that can be described as an Understanding-first approach.
Nor am I suggesting we have students try to determine the equations of difficult straight lines before they have worked with gradients and ordered pairs.
Nor am I suggesting there is NO place for compartmentalising when teaching mathematics.
What I am suggesting, and with conviction, is that the traditional, compartmentalised approach, by default, makes the learning of mathematics more difficult than it needs to be. I’m suggesting that, by default, compartmentalising the teaching of mathematics has more students spending more time in more lessons NOT understanding the work they have been tasked to complete - compared to a less-compartmentalised approach.
To appreciate why compartmentalising complicates the learning of mathematics - rather than making it more accessible - one needs to experience the alternative. The alternative is to present mathematics in a more unified way, a way that can be described as an Understanding-first approach.
|
One of the key reasons a compartmentalised approach makes learning mathematics more difficult lies in the step-by-step nature of the instruction. A compartmentalised approach can be described as a Procedures-first approach, one in which mathematics is primarily taught through the teaching of procedures with the belief that understanding follows the sufficient practice of procedures.
However, a Procedures-first approach, by default, has students working with procedures without first understanding the concepts upon which the procedures are based. When we expect students to work through procedures without first allowing them to explore and understand the related concepts, we run the risk of turning mathematics into, for many students, a meaningless, boring, unattainable chore. |
What’s the alternative?
In this article I provide insight into, and examples of, a non-compartmentalised (unified) approach to teaching mathematics.
To watch a teacher explain her success when implementing a non-compartmentalised, straight-line graphs unit with the ‘bottom’ year nine class (a highly-structured, student-centred. conceptually-based approach) watch 4-minutes of this video from the 25-minute point.
Finally, these two online courses provide a roadmap for teachers to adopt an Understanding-first, Procedures-second approach (also referred to as a highly-structured, (somewhat) student-centred, conceptually-based approach).
To watch a teacher explain her success when implementing a non-compartmentalised, straight-line graphs unit with the ‘bottom’ year nine class (a highly-structured, student-centred. conceptually-based approach) watch 4-minutes of this video from the 25-minute point.
Finally, these two online courses provide a roadmap for teachers to adopt an Understanding-first, Procedures-second approach (also referred to as a highly-structured, (somewhat) student-centred, conceptually-based approach).
Related Articles
Why Students Need To Understand The Concept Before We Teach Them The Procedure - here
Let's stop forcing our mathematics students to play the memory game! - here
Why We Need An Understanding-first, Procedures-second Mindset When Teaching Mathematics - here
Too Many Kids Hate Maths - here
The Understanding-first, Procedures-second Approach In Action - Three Examples - here
Could These Four Aspects Of Mathematical Understanding Change The Way We Teach Maths? - here
70% Of Capable Students Are Failing Mathematics. What Can We do? - here
Call to Action
What about you? Have you thought about compartmentalisation and whether or not there is a better way?
Do you strive to present mathematics in a more unified, less compartmentalised manner?
NOTE: Create a Hyvor account before commenting (click LOGIN) -that way you'll be notified of replies and you won't be anonymous.
If you don't create an account, please state your name at the start of your comment. Thanks.
Do you strive to present mathematics in a more unified, less compartmentalised manner?
NOTE: Create a Hyvor account before commenting (click LOGIN) -that way you'll be notified of replies and you won't be anonymous.
If you don't create an account, please state your name at the start of your comment. Thanks.
Some Past Comments